Achegándonos ao LHC
| A Radiación Sincrotrón é, dun xeito sinxelo, radiación procedente de partículas cargadas que se moven a velocidades relativistas baixo un campo magnético uniforme. É o equivalente da radiación ciclotrón e toma o nome dos aceleradores relativistas. Cando os ciclotróns son suficientemente poderosos para lanzar ás partículas preto da velocidade da luz, a frecuencia orbital desa partícula cambia. Precísase sincronizar os parámetros do acelerador para que se adapte a esos cambios. Temos entón un acelerador sincrotrón. As partículas sometidas a unha traxectoria permanentemente curvada emiten radiación: a radiación sincrotrón. |
|
Instalacións que usan a radiación sincrotrón están en funcionamiento en diversos lugares do mundo como fontes moi valiosoas de fotóns UV e R-X, para investigación estrutural.
No universo créanse electróns relativistas que son atrapados en campo magnéticos diversos. Os obxectos cósmicos que emiten radiación sincrotrón nesas condicións son moi interesanteno campo da Astrofísica.
Sen embargo, nos aceleradores de partículas circulares, como o LHC, esta radiación supón un serio problema. As partículas cargadas viaxando en traxectorias curvadas emiten esta radiación, e xa que logo perden enerxía. Ademais, esa radiación constitúe un problema para todos os sistemas do acelerador (en particular a crioxenia) e tamén desde o punto de vista da seguridade radiolóxica.
As cavidades RF deben restituir esa enerxía perdida polas partículas.
Por revolución, a potencia perdida é:
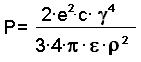
(donde ρ é o radio)
É importante destacar que dado que ϒ = E/m0·c2, os electróns perden enerxía 1013 vecesmáis rápido que os protóns. Esta é unha das razóns de que no LHC corran protóns, e que o posible acelerador de electróns do futuro (CLIC ou ILC) será un acelerador lineal.
Obteñamos ρ en función doutros parámetros do acelerador e sustituamos o seu valor na expresión anterior:
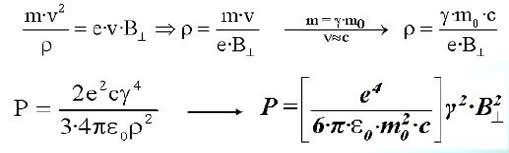
con B = 8,33 T e ϒ = 7640 temos: P = 1,93·10-11 W
A enerxía perdida por volta (1 s = 11245 voltas), é dicir a chamada Synchrotron radiation loss/turn:
1,93·10-11/11245 = 1,72·10-15 J/volta
ou, en keV: ~ 10 keV/voltaDoutra banda, para cada feixe: Pbeam = 1,90·10-11 ·1,15·1011 · 2808 ⇒ Pbeam ≃ 6135 W
Finalmente, a enerxía perdida por segundo e metro é:
O xeito de reducir o número total de partículas sen comprometer a luminosidade consiste en incrementar o espazo entre bunches -bunch spacing- (utilizar menos bunches) e compensar esta reducción aumentando o número de protóns en cada bunch.
|
AUTORES Xabier Cid Vidal, Doctor en Física de Partículas (experimental) pola Universidad de Santiago (USC). Research Fellow in experimental Particle Physics no CERN, desde xaneiro de 2013 a decembroe de 2015. Estivo vencellado ao Depto de Física de Partículas da USC como becario "Juan de la Cierva", "Ramon y Cajal" (Spanish Postdoctoral Senior Grants), e Profesor Contratado Doutor. Desde 2023 é Profesor Titular de Universidade nese Departamento (ORCID). Ramon Cid Manzano, foi catedrático de Fïsica e Química no IES de SAR (Santiago - España), e Profesor Asociado no Departamento de Didáctica de Ciencias Experimentais da Facultade de Educación da Universidad de Santiago (España), ata o seu retiro en 2020. É licenciado en Física, licenciado en Química, e Doutor pola Universidad de Santiago (USC).(ORCID). |
CERN CERN Experimental Physics Department CERN and the Environment |
LHC |
NOTA IMPORTANTE
Toda a Bibliografía que foi consultada para esta Sección está indicada na Sección de Referencias
© Xabier Cid Vidal & Ramon Cid - rcid@lhc-closer.es | SANTIAGO |